The Pascaline of Blaise Pascal
The Pascaline (or roue Paschaline, as it is called in 17th century) of the great french scientist Blaise Pascal was for long time considered as the first mechanical calculator in the world, as the Rechenuhr of Schickard was not widely known until 1950s. Pascal had no access to the correspondence of Kepler, so he is hardly likely to know anything about Schickard. It is more likely Pascal to have read the Annus Positionum Mathematicarumof Dutch Jesuit-mathematician Jan Ciermans (1602-1648), who mentioned in this book, that he created a mechanical calculator with iron wheels, suitable for multiplication and division.
So, how it all began?
In 1639 Étienne Pascal, the father of Blaise Pascal (see biography of Pascal) was appointed by the Cardinal de Richelieu as Commissaire député par sa Majesté en la Haute Normandie (financial assistant to the intendant Claude de Paris) in Rouen, capital of the Normandy province. Étienne Pascal arrived in the city of Rouen in January 1640. He was a meticulous, forthright and honest man, and spent a considerable amount of his time completing arithmetic calculations for taxes. The task of calculating enormous amounts of numbers in millions of deniers, sols and livres necessitated ultimately the help of his son Blaise and one of his cousins' son, Florin Perrier, who would soon marry Blaise's sister Gilberte. The young men used initially only manual calculations and abacus, but in 1642 the Blaise started to design a calculating machine. The first variant of the machine was ready next year, and the young genius continued his work on improving his calculating machine.
In his later pamphlet (Advis necessaire) Pascal asserted: ...For the rest, if at any time you have thought of the invention of machines, I can readily persuade you that the form of the instrument, in the state in which it is at present, is not the first attempt that I have made on that subject. I began my project by a machine very different from this both in material and in form, which (although it would have pleased many) did not give me entire satisfaction. The result was that in altering it gradually I unknowingly made a second type, in which I still found incon veniences to which I would not agree. In order to find a remedy, I have devised a third, which works by springs and which is very simple in construction. It is that one which, as I have just said, I have operated many times, at the request of many persons, and which is still in perfect condition. Nevertheless, in constantly perfecting it, I have found reasons to change it, and finally recognizing in all these reasons, whether of difficulty of operation, or in the roughness of its movements, or in the disposition to get out of order too easily by weather or by transportation, I have had the patience to make as many as fifty models, wholly diff"erent, some of wood, some of ivory and ebony, and others of copper, before having arrived at the accomphshment of this machine which I now make known. Although it is composed of many different small parts, as you can see, at the same time it is so solid that, after the experience of which I have spoken before, I assure you that all the jarring that it receives in transportation, however far, will not disarrange it.
First several copies (certainly made by a local clockmaker in Rouen, it was a time when clocks and clockwork were celebrated, with even the Universe being considered, at least metaphorically, as being a form of clockwork) of the machine didn't satisfied the inventor. Meanwhile, in 1643, it happened an event, which almost manage to give up Pascal from the machine. A clockmaker from Rouen dared, (according to the words of the offended inventor, who named no name—whether he knew it is unknown), to make a beautiful, but absolutely useless for work copy of the machine. Let's look again how is describing this event Pascal himself in his pamphlet (Advis necessaire):
...Dear reader, I have good reason to give you this last advice, after having seen with my own eyes a wrong production of my idea by a workman of the city of Rouen, a clockmaker by profession, who, from a simple description which had been given him of my first model, which I had made some months previously, had the presumption, to undertake to make another; and what is more, by another type of movement. Since the good man has no other talent than that of handling his tools skillfully, and has no knowledge of geometry and mechanics (although he is very skillful in his art and also very industrious in many things which are not related to it), he made only a useless piece, apparently true, polished and well filed on the outside, but so wholly imperfect on the inside that it was of no use. Because of its novelty alone, it was not without value to those who did not understand about it; and, notwithstanding all these essential defects which trial shows, it found in the same city a place in a collector's cabinet which is filled with many other rare and curious pieces. The appearance of that small abortion displeased me to the last degree and so cooled the ardor with which I had worked to the accomphshment of my model, that I at once discharged all my workmen, resolved to give up entirely my enterprise because of the just apprehension that many others would feel a similar boldness and that the false copies which they would produce of this new idea would only ruin its value at its beginning and its usefulness to the public. But, some time afterward, Monseigneur le Chancelier, having deigned to examine my first model and to give testimony of the regard which he held for that invention, commanded me to perfect it. In order to eliminate the fear which held me back for some time, it pleased him to check the evil at its root, and to prevent the course it could take in prejudicing my reputation and inconveniencing the pubhc. This was shown in the kindness that he did in granting me an unusual privilege, and which stamped out with their birth all those illegitimate abortions which might be produced by others than by the legitimate alliance of the theory with art.
...Dear reader, I have good reason to give you this last advice, after having seen with my own eyes a wrong production of my idea by a workman of the city of Rouen, a clockmaker by profession, who, from a simple description which had been given him of my first model, which I had made some months previously, had the presumption, to undertake to make another; and what is more, by another type of movement. Since the good man has no other talent than that of handling his tools skillfully, and has no knowledge of geometry and mechanics (although he is very skillful in his art and also very industrious in many things which are not related to it), he made only a useless piece, apparently true, polished and well filed on the outside, but so wholly imperfect on the inside that it was of no use. Because of its novelty alone, it was not without value to those who did not understand about it; and, notwithstanding all these essential defects which trial shows, it found in the same city a place in a collector's cabinet which is filled with many other rare and curious pieces. The appearance of that small abortion displeased me to the last degree and so cooled the ardor with which I had worked to the accomphshment of my model, that I at once discharged all my workmen, resolved to give up entirely my enterprise because of the just apprehension that many others would feel a similar boldness and that the false copies which they would produce of this new idea would only ruin its value at its beginning and its usefulness to the public. But, some time afterward, Monseigneur le Chancelier, having deigned to examine my first model and to give testimony of the regard which he held for that invention, commanded me to perfect it. In order to eliminate the fear which held me back for some time, it pleased him to check the evil at its root, and to prevent the course it could take in prejudicing my reputation and inconveniencing the pubhc. This was shown in the kindness that he did in granting me an unusual privilege, and which stamped out with their birth all those illegitimate abortions which might be produced by others than by the legitimate alliance of the theory with art.
Later on, however, friends of Pascal presented to the Chancellor of France, Pierre Seguier (1588–1672), a prototype of the calculating machine. Seguier admired the invention and encouraged Pascal to resume the development. In 1645 Pascal wrote a dedicatory letter at the beginning of his pamphlet (the above mentioned Advis necessaire) describing the machine (actually advertising the machine, as almost nothing is mentioned about its construction and operation) (see the letter and the pamphlet of Pascal), and donated a copy of the machine to the Chancellor (still preserved in CNAM, Paris). The text concluded that the machine could be seen in operation and purchased at the residence of Prof. Gilles de Roberval. This is the only preserved description of the device from the inventor.
The Chancellor Seguier continued to support Pascal and on 22 May, 1649, by royal decree, signed by Louis XIV of France, Pascal received a patent (or privilege as it then was called) on the arithmetical machine, according to which the main invention and movement is this, that every wheel and axis, moving to the 10 digits, will force the next to move to 1 digit and it is prohibited to make copies not only of the machine of Pascal, but also of any other calculating machine, without permission of Pascal. It is prohibited for foreigners to sell such machines in France, even if they are manufactured abroad. The violators of the privilege will have to pay penalty of 3 thousand livres (see the Privilege of Pascal).
The privilege again (as the Advise) mentions that Pascal has already produced fifty somewhat different prototypes. Moreover, the patent was awarded gratis and did not specify an expiration time, which was rather unusual affair. It seems Pascal was an authentic favorite of the french crown :-)
The Chancellor Seguier continued to support Pascal and on 22 May, 1649, by royal decree, signed by Louis XIV of France, Pascal received a patent (or privilege as it then was called) on the arithmetical machine, according to which the main invention and movement is this, that every wheel and axis, moving to the 10 digits, will force the next to move to 1 digit and it is prohibited to make copies not only of the machine of Pascal, but also of any other calculating machine, without permission of Pascal. It is prohibited for foreigners to sell such machines in France, even if they are manufactured abroad. The violators of the privilege will have to pay penalty of 3 thousand livres (see the Privilege of Pascal).
The privilege again (as the Advise) mentions that Pascal has already produced fifty somewhat different prototypes. Moreover, the patent was awarded gratis and did not specify an expiration time, which was rather unusual affair. It seems Pascal was an authentic favorite of the french crown :-)
It seems later Pascal wanted to manufacture his machines as a full scale business enterprise, but it proved too costly, and he didn't manage to make money from this privilege. It's not known how many machines were sold but the total was probably no more than ten or fifteen. Price may have been the main issue here, though accounts vary significantly, from the Jesuit mathematician François's 100 livres to Tallemant de Réaux's 400 livres and Balthasar Gerbier's 500 livres (let's mention, that 100 livres were enough to keep a seventeenth-century Frenchman in modest comfort for a year).
Pascal continued to experiment, constructing a lot of variants of the machine (later on called the Pascaline or Pascalene). He worked so hard on this machine, it is said, that his mind was disturbed (avoir latête démontée) for the next three years. According to his sister Gilberte, the young inventor's exhaustion did not come from the labor he put into designing the machine, but rather in trying to make the Rouen artisans understand what it was all about.
Pascal decided to test the reliability of the machine, sending a copy to a long journey with carriage (from Rouen to Clermont and back, some one thousand kilometers) and the machine returned in perfect condition. Later he wrote: "Although [the Pascaline] is composed of many different small parts, as you can see, at the same time it is so solid that, after the experience of which I have spoken before of ['carrying the instrument over more than two hundred and fifty league of road, without its showing any damage'], I assure that the jarring that it receives in transportation, however far, will not disarrange it.
Actually, the mechanism for tens carry is not very reliable and machine have to be in position, near to horizontal, in order to work reliable, and sometimes, a hit to the box can cause unwanted carry to be performed.
The Pascaline soon become well-known in France and abroad. The first public description was in 1652, in the newspaper Muse Historique. The machine was demonstrated to the public in Paris. Pierre de Ferval, a family friend and a mathematics professor at the Royal College of France, agreed to demonstrate the device to prospective customers in his apartment at the College Maitre Gervais every Saturday morning and afternoon. Pascal went to work writing advertising flyers for the invention and asked a friend, the poet Charles Vion Dalibray, to compose a publicity sonnet. The Polish queen Marie Louise de Gonzague, a high-ranking and keen patron of sciences, asked to buy two copies. Another fan of sciences, Swedish queen Christina desired a copy to be granted to her. Pascal satisfied their desire (the device for queen Christina was sent together with a short manual), but soon after this lost his interest and abandoned his occasions with the calculation machine until the end of his short life.
A Pascaline from 1652 (© Musée des Arts et Métiers, Paris)
From some 50 constructed Pascalines, only 8-9 survived to the present day, and can be seen in private or museum collections (4 in CNAM, Paris, 2 in a museum in Clermont, and several in private collections, e.g. in IBM).
First copies of the machine were with five digital positions. Later on Pascal manufactured machines with 6, 8, and even 10 digital positions. Some of the machines are entirely decimal (i.e. the scales are divided to 10 parts), others are destined to monetary calculations and have scales with 12 and 20 parts (according to french monetary units: 1 sol = 12 deniers, 1 livre = 20 sols).
The dimensions of brass box of the machine (for 8 digital positions variant) is 35.1/12.8/8.8 cm. The input wheels are divided by 10, 12 or 20 spokes, depending of the scale. The spokes are used for rotating of the wheels by means of a pin or stylus. The stylus rotates the wheel until it get to an unmovable stop, fixed to the lower part of the lid. The result can be seen in the row of windows in upper part, where is placed a plate, which can be moved upwards and downwards, allowing to be seen upper or lower row of digits, used for addition or subtraction.
Let's examine the principle of action of the mechanism, using the lower sketch.
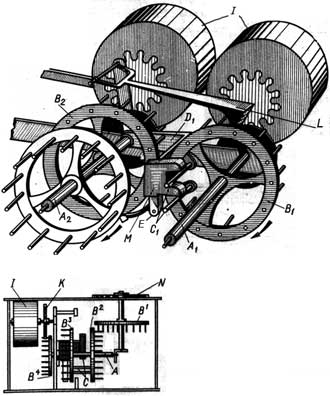
A sketch of the calculating mechanism of Pascaline
The input wheels (used for entering of numbers) are smooth wheels, across which periphery are made openings. Counter-wheels are crown-wheels, i.e. they have openings with attached pins across periphery.
The movement is transferred from the input wheel (marked with N in the sketch), which can be rotated by the operator by means of a stylus, over the counter, which consists of four crown-wheels (marked with B1, B2, B3 and B4), pinion-wheel (K), and mechanism for tens carry (C), to the digital drum (I), which digits can be seen in the windows of the lid.
The tens carry mechanism (called by Pascal sautoir), works in this way:
On the counter-wheel of the junior digital positions (B1) are mounted two pins (C1), which during the rotating of the wheel around its axis (A1) will engaged the teeth of the fork (M), placed on the edge of the 2-legs rod (D1). This rod can be rotated around the axis (A2) of the senior digital position, and fork has a tongue (E) with a spring. When during the rotating of the axis (A1) the wheel (B1) reach the position, according to the digit 6, then pins (C1) will engaged with the teeth of the fork, and in the moment, when the wheel moves from 9 to 0, then the fork will slide off from the engagement and will drop down, pushing the tongue. It will push the counter wheel (B2) of the senior position one step forward (i.e. will rotate it together with the axis (A2) to the appropriate angle. The rod (L), which has a special tooth, will serve as a stop, and will prevent the rotating of the wheel (B1) during the raising of the fork. The tens carry mechanism of Pascal has an advantage, compared e.g. to this of Schickard's Calculating Clock, because it is needed only a small force for transferring the motion between adjacent wheels. This advantage however is paid by a some shortcomings—during the carrying is produced a noise, and if the box is hit, may occur unwanted carrying.
On the counter-wheel of the junior digital positions (B1) are mounted two pins (C1), which during the rotating of the wheel around its axis (A1) will engaged the teeth of the fork (M), placed on the edge of the 2-legs rod (D1). This rod can be rotated around the axis (A2) of the senior digital position, and fork has a tongue (E) with a spring. When during the rotating of the axis (A1) the wheel (B1) reach the position, according to the digit 6, then pins (C1) will engaged with the teeth of the fork, and in the moment, when the wheel moves from 9 to 0, then the fork will slide off from the engagement and will drop down, pushing the tongue. It will push the counter wheel (B2) of the senior position one step forward (i.e. will rotate it together with the axis (A2) to the appropriate angle. The rod (L), which has a special tooth, will serve as a stop, and will prevent the rotating of the wheel (B1) during the raising of the fork. The tens carry mechanism of Pascal has an advantage, compared e.g. to this of Schickard's Calculating Clock, because it is needed only a small force for transferring the motion between adjacent wheels. This advantage however is paid by a some shortcomings—during the carrying is produced a noise, and if the box is hit, may occur unwanted carrying.
The wheels of the calculating mechanism are rotating only in one direction and there are not intermediate wheels provided (designated to reverse the direction of the rotation). This means, that the machine can work only as a adding device, and subtraction must be done by means of a arithmetical operation, known as complement to 9. This inconvenience can be avoided by adding of additional intermediate gear-wheels in the mechanism, but Pascal, as well as all next inventors of calculating machines (Leibniz,Lepine, Leupold, etc.) didn't want to complicate the mechanism and didn't provide such possibility.
The rotating of the wheels is transferred via the mechanism to the digital cylinders, which can be seen in the windows (see the photo below).
A view to the digital cylinders of Pascaline
On the surface of cylinders are inscribed 2 rows of digits in this way, that the pairs are complemented to 9, for example if the upper digit is 1, the lower is 8. On the lid is mounted a plate (marked with 2 in the lower sketch), which can be moved upwards and downwards and by means of this plate, the upper row of digits must be shown during the subtraction, while the lower one—during the addition. If we rotate the wheels, we will notice that digits of the lower row are changing in ascending order (from 0 to 9), while the digits of the upper row are changing in descending order (from 9 to 0).
Zeroing of the mechanism can be done by rotating of the wheels by means of the stylus in such way, that between the two starting spokes (marked on the wheel) to be seen 9 (see the lower sketch). In this moment the digits of the lower row will be 0, while the upper digits will be 9 (or 12 or 20, for sols and deniers) (see the lower sketch). The manuscriptUsage de la machine (this is the earliest known manuscript for Pascaline, from the 18th century. The first part of this document is a manual for an accountant pascaline and describes how to perform the addition, the subtraction, the multiplication and the division.) gives the following method:
"before starting a calculus, you shift the sliding cover who lays over the display windows toward the edge of the machine. Then you have to set the marked spokes in order to read "0" on all the drums. This is done by setting the stylus in between the spokes that are marked with white paper and by turning the wheel until the needle stops it. This brings for each wheel the highest digit the drum can have, that is to say "9" for all the wheels devoted to the "Livres", "19" on the "sols" wheel and "11" on the "deniers" wheel. Then you you turn the last wheel on the right of only one position [...] afterwards all the drums will display "0"."
"before starting a calculus, you shift the sliding cover who lays over the display windows toward the edge of the machine. Then you have to set the marked spokes in order to read "0" on all the drums. This is done by setting the stylus in between the spokes that are marked with white paper and by turning the wheel until the needle stops it. This brings for each wheel the highest digit the drum can have, that is to say "9" for all the wheels devoted to the "Livres", "19" on the "sols" wheel and "11" on the "deniers" wheel. Then you you turn the last wheel on the right of only one position [...] afterwards all the drums will display "0"."
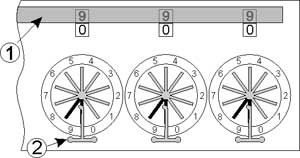
Zeroing of the mechanism of Pascaline
An instruction for work with the machine from Pascal didn't survived to the present day, so in different sources are described different ways of manipulation. I will describe a way, which is optimal as number of operations, needed for performing of calculations. To use this way however, the operator must know the multiplication table (during the multiplication operation), and to be able to determine a complement to 9 for digits (for division and subtraction). This is an easy task even for 8 years old child now, but not for the men of 17th century. Of course, the calculations can be done without following the two upper mentioned requirements, but it will be necessary more attention and additional movements of the wheels.
First, let's make an addition, for example 64 + 83. We have to put the stylus between the spokes of the units wheels, against 4 and to rotate the wheel to the stop. In the lower row of windows (the upper was hide by the plate) we will see 4. Then we rotate the wheels of the tens by the same way to 6. Then we have to enter the second addend, 83, and we will see the result, 147, meanwhile one carry will be performed.
The subtraction will be a little more difficult and will require not only rotating, but some mental work. Let's make, for example, 182–93.
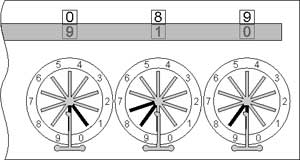
After zeroing of the mechanism (to see 000 in the lower windows), the plate of the windows must be moved in lower position and at this moment in the windows can be seen the number 999. Then the minuend is entered as a complement to 9, i.e. the units-wheel is rotated for 7, the tens-wheel for 1, and hundreds-wheel to 8 (the complement to 9 of 182 is 817). As the upper row of digits actually is moved to descending order, thus we have made a subtraction 999-817 and the result is 182 (see the lower sketch).
Subtraction with the Pascaline (first phase)
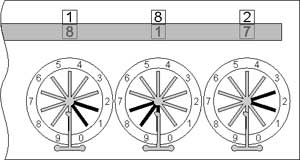
Then must be entered the subtrahend (93), making a subtraction 182–93 (during rotating of the wheels two carries will happen—during the entering of the units (3), the units wheel will come to 9, and a carry to the tens-wheel wheel will be done, moving the tens-wheel to 7; then during the entering of 9 to the tens-wheel, it will be rotated to 8, and a carry will be transferred to the hundreds-wheel, making it to show 0). So, we have the right result 182–93=089 (see the lower sketch).
Subtraction with the Pascaline (second phase)
It wasn't that difficult, but the operator must be able to determine complement to 9 of a number.
To be able to use the fastest way for multiplication, the operator must know (or use) a multiplication table. Let's make the multiplication 24 x 38. First we have to multiply (mentally or looking at the table) units of the multiplicand to the units of the multiplier (8 x 4 = 32) and enter the result 32 in the mechanism (see the lower sketch).
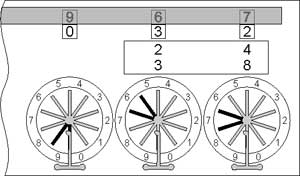
Multiplication with the Pascaline (first phase)
Then we have to multiply units of the multiplier to the tens of the multiplicand (8 x 2 =16), but to enter the result (16) not in the right most digital positions (for units and tens), but in the next (the positions for tens and hundreds). This we will have the result 192 (32 + 160) (see the lower sketch).
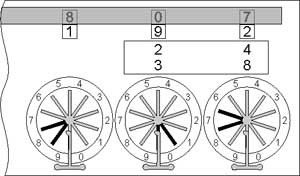
Multiplication with the Pascaline (second phase)
Then we have to repeat the same operation for multiplication of the units of the multiplicand to the tens of the multiplier (3 x 4 =12) and for multiplication of the tens of the multiplier to the tens of the multiplicand (3 x 2 = 6), entering the intermediate results into wheels of tens and hundreds (12), and into the hundreds and thousands (06). We have the right result (912) (see the lower sketch).
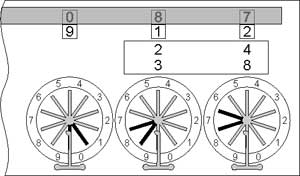
Multiplication with the Pascaline (third phase)
The division with the Pascaline can be done in the way, similar to the manual division of the numbers—first we have to separate the dividend to 2 parts (according to the value of the divisor). Then we have to perform consecutive subtractions of divisor from the selected part of dividend, until the remainder will become smaller then the part. In this moment we have to write down the number of subtractions, this will be the first digit of the result. Then we have to attach to the remainder (if any) 1 or more digits from the remained part of the dividend and to start again the consecutive subtractions, until we receive the second digit of the result and to continue this operation again and again, until the last digit of the dividend will be used. At the end we will have the remainder of the division in the windows, while the result will be written.
It's quite obvious, that the work with the Pascaline is not very easy, but the machine is completely usable for simple calculations.
Some people then almost suggest Pascal was in possession of some kind of magical powers during his work on Pascaline. e.g. in Entretien avec M. de Sacy: It was common knowledge that [Pascal] seemed able to animate copper, and to give to brass the power of thought. Little unthinking wheels, each rimmed with then ten digits, were so arranged by him that they could give accounts even to the most reasonable persons, and he could in a sense make dumb machines speak.
Pierre Petit (1617–1687)—a French scholar and an inventor of a tool with Napier's rodswrote: I find that since the invention of logarithms and rabdology, nothing of significance occurred regarding the practice of numbers other than Monsieur Pascal's instrument. It is a device truly invented with as much success and speculation as his author has intelligence and knowledge. It consists, however, in a number of wheels, springs, and movements, and one needs the head and hands of a good clockmaker to understand how it works and to manufacture it, as well as the skills and knowledge of a good arithmetician to operate it. [For all these reasons], one fears that its use will never become widespread, and that instead of being employed in financial bureaux and regional administrations to calculate taxes, or in merchant offices to compute their rules of discount and company, [the machine] will be stored in cabinets and librairies, there to be admired.
Admittedly, not all impressions from Pascal's contemporaries were positive. Some was unfavorable, such as the October 1648 letter of the English gentleman traveller Balthasar Gerbier to Samuel Hartlib. Gerbier came upon Pascaline not long after a model in wood was finished, and thought it resembled something invented in England 30 years earlier. (Gerbier most likely meant William Pratt's Arithmetical Jewel from 1616, a simple calculating instrument, that was nothing more than a variant of the common abacus). Gerbier though found many problems with the Pascaline.
First, its user had to be knowledgeable in arithmetic, which ran contrary to Pascal's rhetorical stance. Multiplications and divisions were complicated and it even took two Pascalines to make a simple rule of three. Gerbier also found Pascaline rather big (two foot in length, 9 inches broad), heavy, difficult to move, expensive (50 pistoles, or 500 livres) and useless to anyone who would like to learn the art of arithmetic. In other words, Gerbier did not admire this mechanical contraption supposed to "think" by itself. He ended his letter to Hartlib quoting a former ruler of Netherlands: Infine a Rare Invention farre saught, and deare baught: putt them jn the Storre house was the old Prince of Orange wont to saye and lett us proceede on the ordinary readdy way.
